Today's Slides and Homework: February 29

Here they are ...

| View | Upload your own

Scribe Post

Today's Slides and Homework: February 28

Here they are ...

| View | Upload your own

Scribe Post

Hi Everyone! Sorry for it being late! The first part of class in the morning we went over our homework from last night. After that we had a warm up to our next topic in Probability “The Fundamental Principal of Counting.”

Warm Up….
Question # 1
Find the probability of flipping three pennies and getting at lest 1 heads.

The first penny would be either heads or tails; the second would be also heads or tails etc. The chance of getting either one on any of the pennies on the three times would be ½., because there are 2 sides to a penny.

*Remember to watch how the question is worded.
Ex. 1. Heads AND Heads AND Heads mean multiplied.
2. Heads OR Heads mean add*



Question # 2

How Many Ways

A.) The café special for lunch offers a choice between two main courses (hamburger or chicken burger) and three different drinks. The “meal deal” allow you to pick one each. How many different “meal deal” are there?
*2 meals times the # of meal deals = 6 diff. ways.

B.) What if they decide to throw in a choice of fries, spicy fries or plain chips. How many “meal deals” are there now?
*Now you add the fries, spicy fries & plain chips on.

*Now you just add the 3 more diff meals to the first two. Witch now = to 18 diff. ways.

Now this is what the warm ups got us warmed up for…….

“The Fundamental Principal of Counting”

If there are M ways to do a first thing & N ways to do second thing then there are M * N ways to do both things.

Ex. Any one of 4 ties can be matched with any one of 3 shirts, how many shirts & ties combinations are possible?
* 4 ties times 3 shirts = 12 diff. ways to find combinations.

What if there are also 2 different pairs of pants that can be matched with all the shirts and ties, how many different “outfits are there now?

Now you try……

1.) How many 4 digit #’s are there if the same digit CANNOT be used twice?

* The First # can’t start with 0.

2.) How many 4 digit #’s are there if the same CAN be repeated?

* The same rule applies here can’t start with 0.

3.) How many 4 digit EVEN # CANNOT be used twice?

















Well I think that is about it….I hope you guys/ gal’s understand what I wrote. The next scribe is…….Mercee

Today's Slides and Homework: February 27

Here they are ...

| View | Upload your own

Scribe Post - Pascal's Triangle

Hello Boys & Girls,
Well the first math class (this morning) we had a pep rally. The beginning of afternoons class we went over last nights homework. Then we went over Pascals Triangle.

We started off with something alittle simple. We went over a number triangle.


- To get this trianle of numbers, you see that all the numbers on the right and left side are all the number 1.
- To get the middle numbers you add the numbers above it. So for the 3rd row you add 1+1=2.
- The 4th row to get the 3's you add 1+2=3.
- The 5th you add 1+3=4 , 3+3=6, 3+1=4
and so on.



We also went over many different ways to find patterns in Pascals Triangle.

The Pink line is just showing the its the numbers 1-10.
The BLUE Lines represent the "hockey stick".
-When you add 1,3,6, &10 together you get 20.
-When you add 1,6, & 21 together you get 28.

The GREY line is how many "dots" would make a triangle. Example:
< -----




Also, if you put
11^0 = 1
11^1 = 11
11^2 = 121
11^3 = 1,331
11^4 = 14,641


Another way of using Pascal's Triangle is in a word problem. Like this :



Home = top left corner
School = bottom right corner
and the other is post office.



In that problem it asks how many ways to get to the pst office from home. By using pascal's triangle, we figured that there are 3 ways to get from home to the post office.

ALRIGHTY, well i think thats all, hopefully it helps. (:
Next to scribe is ....... Vanessa

Today's Slides and Homework: February 26

Here they are ...

| View | Upload your own

Scribe Post : Probability

Hey guys.. ok so today we worked more on probability specifically theoretical and experimental probability. As a recap:

Experimental Probability is the chance of something happening based on completing an experiment.
Ex: Tossing 3 pennies, using heads to represent boys and tails for girls, then repeating the toss 10 times, representing a family with three children.

Theorectical Probability is the chance of something happening based on realistic circumstances.
Ex.When having a child, there are only two outcomes. Either the child is a girl or boy, therefore there is a 50% chance it will be a girl and 50% chance it will be a boy. For the second child, there are no restrictions as to wether the child will be a girl or boy, so then once again the chance of having a girl remains 50% and for a boy 50% aswell.

Today we learned that we can easily use a tree diagram to help us find outcomes. For example using the example as stated under the theorectical definition:

Remember: inorder for it to be easily followed or understandable, the diagram must be expressed neatly.

So if you follow the branches of the tree diagram, you would get outcomes such as:

B B B

B B G

B G B

B G G - exactly 2 girls outcome #1

G B B

G B G - exactly 2 girls outcome #2

G G B - exactly 2 girls outcome #3

G G G

For this example, it asks how many outcomes consist of exactly 2 girls. The answer would be "3 out of 8"

Today we learned how to calculate probability on our TI-83 which we would call stimulating binomial experiments (number of trials, probability of success, number of stimulations). So using the example: In a family of three children, what is the probability that 2 of the children will be girls? We calculated it in class.

So on your TI-83 follow step by step intructions:
1. select [MATH]
2. slide over to [PROB]
3. select [randBin] (random binomial experiment)
4. type in (1, 3/8,40)
- 1 represents the outcome for success.
- 3/8 represents the theorectical probability of success
- 40 represents the # of times the experiement is repeated
5. click [ENTER]
- this will calculate the outcome
- "1" representing successes and "0" representing failures.
Instead of counting the number of time the experiment is successful, it can be calculated throught the calculator, so with #5 of the the steps above still on your screen continue the following:
6. select [STO] [2nd] [L1]
- this will store the information in List 1 (L1)
7. select [2nd] [STAT]
8. select [MATH] slide down to [sum] [2nd] [L1]
- this will calculate of the successes or all the failures.

Like Mr. K said in class informfation such as the information we entered into the calculator: (1, 3/8, 40) you will need to know how to calculate for yourself for test, quizzes and the exam.

ok well think that is it. See ya later!
The Scribe for tomorrow will be Melissa S.

Today's Slides and Homework: February 25

Here they are ...

| View | Upload your own

Scribe Post

Sorry guys my internet was down yesterday but even so, i sure wether or not there was a purpose in my scribe because all we did yesterday in the morning was work on a review, then Mr. Rekrut gave us the answer key in the afternoon period.
hm... Mr. Kuropatwa when you read this can you get back to me please!

BOB

Hey guys, goodluck on the unit test tomorrow, we can do it!!

For me specially, i cleared out most of my muddiest points tonight already. All in all, i think that this unit was piece-easy. It took some time for us to get some of the explanation, but in the end we seem to have gotten it through our heads. Study well guys, goodluck to us all!

BOB - Matrices

I don't think I had a hard time getting the hang of matrices. For once i feel like I understand what's going on in Math class, haha. Sometimes a question does look tricky, especially on the last worksheet we had to do, but when i looked at the answer sheet and saw that I got the same answer, I realized I was guessing it right. On the two questions that I got wrong, I quickly realized what I did wrong and fized my mistake. After that I double checked with some classmates and in the end we had the same answers. The only advice I have for the rest of you is make sure you read the question more than once, even if you think you know what it's asking. I made the mistake of assuming it was asking the obvious a few times already, and believe me, it makes you feel silly when you see you got it wrong.

BOB

Well the matrices unit was fairly easy except for the transition matrix part and some of the word problems. I know i'm not alone when I say that we had a little bit of difficulty with it. But overall, I was able to work with the other problems smoothly. That's it for now. Good luck on the test tommorow everyone !

BOB - Matrices

WELL, like everyone, matrices was a pretty easy unit for me. I was able to do everything except for the transition matrix. Only the big problem ones. Either than that, im able to do everything else. Hopefully the review will make the transition problems better. SOOOOO good luck on the test thursday. study study study! =)

BOB - MATRICES

Like most, I think the matrices unit it pretty easy it's just the word problems that I have a hard time doing.. but hopefully after the review we get tomorrow or the next day will help clear it all up. hm... so yeah that's it for now.

GOODLUCK EVERYONE!

BOB-Matries

The first unit Matries wasn't that bad. I found it to be easy most of the time, but when it came to the word problems that is where I had a hard time. Other than that it wasn't bad.
Till next time bye for now!

Scribe Post:
February 19 - Introduction to Probability

Im not sure if i am supposed to be the scribe for today since i don't see the last scribes that we're supposed to be posted (unless it's just my computer). But i was told today in class by ickie that i am the next scribe. So just incase, I'll do the scribe for today anyway.


Today we started our second unit which is on Probability.
We learned a variety of language today, with many definitions and some formula's.

Probability: The branch of mathematics that deals with chance.
Sample Space: The set of all possible things that can happen for a given set of circumstances.
ex: Rolling a die 6, .. the sample space would then be {1,2,3,4,5,6}.

Event (E): A subset of the sample space. One particular outcome for a given set of circumstances.
Simple Events: The result of an experimental carried out in 1 step.

Compound Event: The result of an experimental carried out in more than 1 step.

Certain Event: An events whose probability is equal to 1.
Impossible Event: An events whose probability is equal to 0.


Calculating Probability Event:


* The "total number of outcomes" is the sample space.

Probability can be expressed as:
- A ratio
- A decimal
- A fraction
- A percent

**Important: Probability is always a number between 0 and 1!


Complimentary Events:
Is either written as E' or E. The compliment of an event refers to the case where E does not occur.

Ex: H= Drawing a Heart from a deck of 52 cards.
H'(the compliment)= Drawing a card that is not a heart.

Calculating Probability Complimentary:
P(E) + P(E') = 1

so ....
P(E) = 1 - P(E') .... OR .... P(E') = 1 - P(E)


Anyway, I hope that helped you guys alittle bit. If not, there are the slides that Mr. K posted up. Maybe you'll be able to understand it better there.
The next scribe will be christine m.

BOB - Matrices

To be frank, i found the matrices unit to be pretty easy, although you need to read the questions which are sometimes re-worded; for example the word problem some of us had trouble on during our matrices work-shop. All in all i found it pretty easy, just need to study. BTW the Godfather is on.

Today's Slides: February 19

Here they are ...

| View | Upload your own

Today's Slides and Homework: February 15

Here they are ...

| View | Upload your own

The Scribe List

This is The Scribe List. Every possible scribe in our class is listed here. This list will be updated every day. If you see someone's name crossed off on this list then you CANNOT choose them as the scribe for the next class.

This post can be quickly accessed from the [Links] list over there on the right hand sidebar. Check here before you choose a scribe for tomorrow's class when it is your turn to do so.

IMPORTANT: Make sure you label all your Scribe Posts properly or they will not be counted.

Cycle 1

christine m. elaineee Adamson Comp NguyenX Jessie Michelle SeZy

Alvin G. vanessa CJ iam.wil Melissa S Grace David-san

ickie (-1) Chris29 CowMilk . . : : Яέήάή : : . . (+1) mercee Grace H2O Ari5

3.23

BOB...Matrices

When talking about matrices it is all pretty simple, until you get into the word problems. Since i missed both classes on monday, i don't really understand exactly what to do. From working on some in class and having other people sort of explain how they got answers I'm starting to pick up little things her and there. But it's not enough for me to be able to do questions on my own, i still don't have a good enough grasp on the whole concept of it all. I'm just hoping that over the next few classes before the test it can get explained a little bit more, and I will probably have to go in for some extra help. Other than the word problem matrices, it has actually been pretty good.

So This is my "BOB" and I am suppose to write problems I have in math. Matrices are pretty understandable and straight forward, and the only places that gives me trouble is the word problems. Sometimes I would have to solve the word problem before I can get into solving the matrix or matrices. So that would be the the spot that makes the tests troublesome for me, but I am sure that others have the same problem even if they are not this this class. That would be all, so far. Eat your vegetables.

Today's Slides and Homework: February 14

Here they are ...

| View | Upload your own

BOB: MATRICES

I'm think i'm good with everything like the basic matrix and using the diagram to make the route matrix or vice versa but transition matrices give me a hard time. if we are given a problem to do i try and sometimes don't get it then when we have the workshop and we go over it i'm ok again until theres another question to do lol. it takes me a while to figure out the problem... maybe just a little more practice? yep..

BOB

Hello 8)
In this Matrices unit I would have to say that my personal "muddiest point" would be the word problems. Involving what we already know into realistic (sometimes) situations. Most of them are quite simple but there are some that are a bit tricky and confusing at the same time. But usually the tricky questions are the ones that just need to be read over more carefully so I can get it. But good thing we had that review practice at class yesterday, it really did help.
See you all later.

BOB

Since the matrices unit had started, i think that i had little problems like working too fast and then making little mistakes. Every time i tried to explain things in class on the board so everyone could understand a bit better i don't really think i did much help but i tried. I know i will get a good mark on the quiz tomorrow but i have to stay up and finish my homework so that i can be able to understand the transition matrices a bit better. Well that all i have to say now its back to the homework later. OH YEAH Don't forget that if you don't have a initial statement in the question, just make sure you read the questions you have to answer like in todays questions about the Oxford and Cambridge schools.

BOB

The matrices unit for me was pretty okay. The only thing I am having a problem with right now is the Transition Matrices. Other than that I think i'll do fine on the test, even though I used to suffer from blanking out on everything once the test is right in front of me. Haha. I hope that we'll get a chance to review on everything we've done on Matrices, so that I can be well prepared for the test on Thursday.

scribe

I’m not sure if this scribe will be much help but it’ll mostly be based on the question we had for homework last night that we also went over in class.

Enjoy =).

So the question that was given to us in class was:

The annual Oxford - Cambridge boat race, has been rowed regularly since 1839. Using data from 1839 up to 1982, there were 57 Oxford wins and 67 Cambridge wins. If the relationship between the results of a given year and the results of the previous year are considered, the following table can be constructed:

--This means that during the 57 games Oxford played, they won 35 times and lost 22 times.

Giving us: 35 + 22 = 57

--Also during the 67 games Cambridge played, they won 23 times and lost 44 times.

Giving us: 23 + 44 = 67

Then part A of the question asked us to “convert the ‘number of wins’ to percentages."

To calculate the number of wins, you divide:

Once you calculated the wins, you also have to convert the number of loses to percentages and to get that, you divide:

After all the calculations are done, we place the numbers back into the matrix and we end up with a transition matrix that looks like this:

Now moving onto part B of the question, which is asking us to find the probability that oxford will win a game this year and the second and third year.

In order to find the probability that Oxford will win this year and the following years, we must construct a state matrix that looks like this:

--The “1” in this state matrix represents the probability that oxford will win.
--The “0” represents the probability Cambridge won't win.

Now that we have both the state matrix and the transition matrix, we can use multiplication to solve part B of the question.


Before I go onto part C, I’ll do part D of the question first because it’s exactly the same as part B. The only difference is that state matrix because now, it looks like this:--This state matrix is different from the previous one we had earlier because now we’re trying to look for the probability Cambridge will win instead of Oxford. Which is why the zero and the one have switched sides.

Now that we have all the information we need, repeat exactly what we did in Part B to part D. Giving us:

Now going back to part C, the question is asking us “over many years, what percentage of games will Oxford win and Cambridge?”

Since the question isn’t asking for when it stabilizes, I just picked a random number like 50, and multiplied it, like so:
And since the question isn’t asking to prove if these are stabilized, I didn’t multiply again by 51 and just left it as is.

Anyways I guess this is the end of my scribe and hopefully it might be some help to some of you. And for tomorrow's scribe, it’ll be ickie

Today's Slides and Homework: February 12

Here they are ...

| View | Upload your own

BOB

I found that the matrices unit was pretty easy. However the problems that I had in this unit was how to solve probability problems with matrices, like the homework we got last night. Also the things that aren't so clear to me and I need a little more explanation, is how to find the intermediates in matrices. Other than that, I think I'll be ready for the test.

February 11,2007 - Transition Matrices

What is Transition Matrices?
- A transition matrix is a square matrix describing the probabilities of moving from one state to another in a dynamic system.
For more info, check out:
http://economics.about.com/library/glossary/bldef-markov-transition-matrix.htm

Example:
In Winnipeg, there is a workforce of 2800 people, with 2500 employed and 800 unemployed. During the course of one year, 20% of the employed workers will lose their jobs and 50% of the unemployed will find jobs.

CLICK THE IMAGE BELOW:

Quotes:

“It isn't that they can't see the
solution.It is that they can't
see the problem.”
-- G. K. Chesterton

Forecast for February 12, 2008(Tuesday)
-11`C with a light snow and a wind of
20km/h.

BTW: Next scribe is YELLOW

Today's Slides and Homework: February 11

Here they are ...

| View | Upload your own

Feb. 08, 2008 (Friday) Connecting Matrices

Hey guys this is Alvin at your service. Sorry for the late blog, I had to work alot this weekend. But here I am now, tired and ready to go!

Feb. 08, 2008 (Friday)

Mr. K gave us a work sheet about Connectivity Matrices. With this worksheet, we used all the things that we already knew about Matricies, and thus excercising and expanding our knowledge towards it. I will show you how to tackle Connecting Matrices by using some of the questions from the worksheet.

The first problem that we were supose to solve was the flightpaths of Atlanta, Boston and Charlotte.

The 3 points are all linked together except for Atlanta and Charlotte. They have no direct flights to eachother. If one wants to go from Atlanta to Charlotte, they will have to go through Boston.

With this, we were assigned to create a matrix that represents the routes between the cities.

The Bunny Hop concept

Before we go any further, we need to understand the "hops". One hop route means that it is a 1 stop flight from 1 city to another. Like for instance a direct flight from Atlanta to Boston or Charlotte to Boston. A two hop

route means that there will be 2 stops in the flight. For example, From Atlanta to Charlotte, you will have to stop in Boston before getting to Charlotte. Or from Charlotte, if you want to go to Atlanta, you will have to stop to Boston before getting there.

One Hop Route!

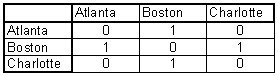This matrix represents a one hop. Look at the first row first column. The number 1 inticate that there is one route between the city in the row and column for that cell. The 0 indicates that there is no route between the two cities.

Two Hop Route!

Tip!

An easy way to figure out the two hop matrix if you have the one hop matrix. You basically just multiply the one hop matrix with the one hop matrix and you will aquire the two hop matrix.

Three Hop Route!

Tip!

You can also get the three hop matrix by mutliplying three one hop matricies.

Closing

I hope that this is helpful to you guys. Sorry if there are any spelling mistakes or any kind of confusion. Contact me via comments and I will correct some errors that you might see on my post. If you still need help about this topic feel free to ask me and I will try my best to help you out.

The scribe for Monday Feb. 11, 2008:

iam.wil

Today's Slides and Homework: February 8

Here they are, Homework begins on slide 10 or download the two jpg files below the slides. You're probably better off viewing the slides in full screen mode online at slideshare. Click the "View" link below the slides here.:

| View | Upload your own

MATRIX MULTIPLICATION

Hey guys!
So, we already learned how to add matrices and multiply matrices by scalars. Today we learned how to solve MATRIX MULTIPLICATION.

What is MATRIX MULTIPLICATION?

- it is the operation of multiplying a matrix with either a scalar or other matrix.

Matrix multiplication is not commutative except in special cases.

Commutative law is when numbers can be added or multiplied in any order

Here is how to do it:



We are asked to multiply matrix A and matrix B.



So first, we have to CHECK the DIMENSIONS. If middle numbers aren't matching we cant multiply them. The middle numbers have to be matching to be able to calculate it. If they are not matching it is not possible to calculate it.
Since they both have 2x2 dimension we dont really need to check the middle number.


-You multiply the rows of matrix A by the columns of matrix B.
-Take the first row of A and the first column of B.
-Then, multiply the first entries and the second entries and then add the two products.
-The sum is one entry of the product matrix AB.
-You just do the same thing when you take the second row of A and the second column of B.

Here are other examples that we did in class today:















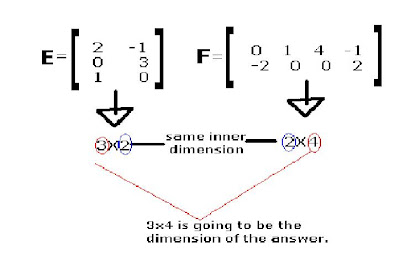
I hope you find my explanation and examples understandable. If you still have problems in this topic please see http://www.purplemath.com/modules/mtrxmult.htm


The next scribe is Chris29.